In two months, Humphrey Lloyd succeeded at observing the predicted light ring of conical refraction. In the early 1830s he used sunlight and a natural crystal. Given today’s wide variety of lasers and synthetic crystals, it is inexplicable that the observation of this fundamental optical effect remains challenging.
Todor Kalkandjiev, Conerefringent Optics SL, Barcelona, Spain
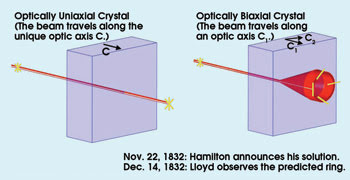
The propagation of light along an optic axis of a crystal depends on the symmetry of the crystal. It is quite well-known that the beam does not change if the crystal is optically uniaxial; however, it undergoes a radical transformation if the crystal is optically biaxial. This is the phenomenon of conical refraction.
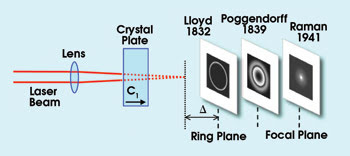
A focused beam passes along the optic axis of a biaxial crystal. The dashed red lines are the imagined continuation of the beam to its focus. The ring plane is shifted by Δ after the focus of the incident beam. Shortly after the ring plane, the light ring splits. Further on, a central point appears, which gets brighter and achieves maximum axial intensity at the position of the focal plane.
Also in the early 1830s, William R. Hamilton found a new property of the Fresnel wave surface, which predicts that, if a narrow beam enters a biaxial crystal along one of its optic axes, it will evolve as a hollow slanted cone, and on the exit surface it will refract as a hollow tube. However, around that time the corpuscular theory of light was dominating, and the discovery’s announcement, in a meeting of the Royal Irish Academy, did not attract much attention. Therefore Hamilton asked Humphrey Lloyd for experimental approval, and two months later Lloyd observed the predicted light ring. This discovery was startling to the scientists and was a triumph for the wave theory.
Lloyd used sunlight and a natural biaxial crystal in his experiment, whereas now there exists a huge variety of lasers and synthetic crystals. Most of the known crystal structures — in fact, about 90 per cent of them — are optically biaxial, such as KTP or LBO. That is why it is strange that the observation of the effect remains challenging, as stated in a recent article by M.V. Berry and M.R. Jeffrey in Progress in Optics (Vol. 50, 2007, pp. 13-50). The authors list only seven known experiments on conical refraction — including Lloyd’s and their own experiment.
In other words, this long-known fundamental optical effect — “among the most beautiful and striking effects arising in crystal optics” (Sir C.V. Raman, 1941) — is practically forgotten today. The ambition of the start-up company Conerefringent Optics SL of Barcelona, Spain, is to bring conical refraction into students’ practices and research photonics labs. The company developed a unique technology to orient crystal elements precisely along an optic axis of a biaxial crystal, and it offers an assortment of elements for conical refraction.
Spatial evolution of the beam
The most specific feature of conical refraction is the light ring appearing at the output. This ring can be seen within a narrow spatial interval, often less than 1 cm, along the beam propagation axis. There is just one position where the light ring has its highest contrast, which is defined as the ring plane.
This is a schematic illustration of the passage of nonpolarized light along an optic axis of a crystal. The light beam does not change in a uniaxial crystal but radically transforms its intensity and polarization distribution in a biaxial crystal. The yellow straight lines represent the polarization state. Every set of two diagonally opposite points of the light tube (right side) are orthogonally polarized.
But there is a misconception about the position of this ring plane in the literature, which assumes that the light ring is formed inside the crystal. Consequently, its observation inevitably would require an imaging lens (optics) behind the crystal, as in all published experimental works until now. However, recent experiments show that the position of the light ring does not depend on the position of the crystal and that ring observation does not require an imaging lens. Actually, the ring plane always is shifted a little behind the focal plane of the incident beam. This shift is given by
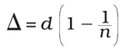
where d is the thickness of the crystal plate, and the refraction index n is the one that the photons “see” during their passage; i.e., the intermediate one n = n2 of the principal refraction indices n1 < n2 < n3.
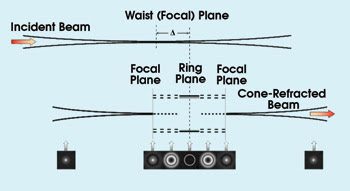
The spatial evolution of an incident Gaussian-like beam and its transformation under the effect of conical refraction are shown. Note the symmetry of the ring plane and the existence of two focal planes.
The above formula also gives a well-known shift of focus when a plane parallel plate is inserted normally in a convergent beam. In ray optics, the formula is obtained by applying Snell’s law and assuming paraxial propagation in a plate made from an isotropic crystal (or glass) or cut from uniaxial crystal perpendicularly to the optic axis. In the case of conical refraction, however, Snell’s law is no longer applicable because of the appearance of a light ring. Nevertheless, the formal substitution of n by n2 gives the observed shift of the ring plane.
Another interesting question is how the beam evolves after the ring plane. It was observed first by J.C. Poggendorff in 1839 that the light ring splits out of the ring plane. Nowadays, the dark ring between both bright rings is named the Poggendorff dark ring. The further beam evolution was determined by Raman 100 years later: A central point appears that gets brighter, and at some position, the axial intensity achieves its maximum. This is the focal plane of the cone-refracted beam. After the focal plane, the beam evolution is more usual and, in the far zone, the intensity profile of the cone-refracted beam coincides with that of the incident beam.
Hence, the cone-refracted beam has a ring plane and a focal plane. What else does it have?
Precision experiments with a HeNe laser beam and a CCD camera show that any two planes that are equidistantly spaced from both sides of the ring plane have equal intensity distributions. Therefore, the ring plane is a plane of symmetry. This property makes obvious the existence of two focal planes of the cone-refracted beam — a distinctive feature that, until now, has been unexploited.
The cascaded conical refraction — the passage of light through two or more consecutive crystals — is another case that is not investigated by the theory. In this one, the exit beam splits into two independent cone-refracted beams. Their rules of transformation cannot be deduced by analogy with known optical effects and suggest potential applications in image processing and laser techniques. The interested reader can find out more about this in a recent paper (Proceedings of SPIE, Vol. 6994, p. 69940B).
What is the future?
Because the effect has been known for nearly 200 years, one might think that there is no room for new ideas or applications. In addition, its rather abstruse theory discourages optical engineers. Thus, geometrical optics defends two kinds of conical refraction: internal and external. The solutions of the diffractive theory describe only one kind of cone-refracted beam that combines features of both the internal conical refraction (propagation along the wave optic axis) and the external one (two focal or “pinhole” planes).
Fortunately, beginning experimental work on conical refraction is now effortless. With one crystal element, a laser pointer and two lenses, most of the historical experiments can be reproduced. With a CCD and the higher resolution of today’s technique, the observer will see details that escaped the discoverers.
To gain access to the market and to new ways of utilization, conical refraction is currently being introduced at the university level. A number of leading European and US universities plan to include experiments on it in students’ curricula this year, with a first step already represented by Barcelona’s Master in Photonics course. Led by professor Carles Pizarro, the students have recently started experimental study.
Several optics groups have started projects to investigate various aspects of conical refraction. Most of them target potential applications. In the end, conical refraction leaves a lasting impression with every researcher who has had the chance to see it.
Contact: Todor Kirilov, Conerefringent Optics SL; phone: +34 938 156 839, e-mail: [email protected].