David Henz, Edmund Optics, Inc.
Creators of infrared systems often encounter
difficulty when trying to select the proper materials for their optical designs.
Part of the challenge is gathering information on the physical properties of IR
materials. The other part is understanding the importance of those properties to
an IR system design.

Zinc selenide IR aspheric lenses from Edmund Optics are uncoated
and provide diffraction-limited focusing performance over a spectral range of 0.6
to 18.0 μm.
The IR region of the electromagnetic spectrum spans 780 nm to
1000 μm. Although the entire IR region is rather large, not all regions are
considered usable because of significant atmospheric absorption bands. Still, there are many applications for the usable IR regions, ranging from missile guidance systems to thermal imaging and from meteorology to medical procedures. Each uses different portions of the IR bands based on specific needs. The optical
materials for these applications also will be different, so developers must examine
the properties of these materials based on their systems’ needs.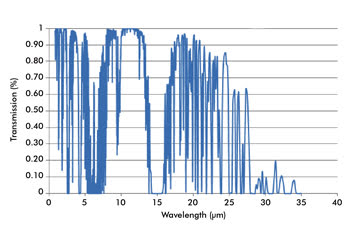
Atmospheric absorption makes sections of the IR spectrum unusable for many applications. The data used here was produced using the program IRTRANS4 and obtained from the UKIRT
(UK Infrared Telescope) website.
Optical properties
Perhaps the most important material properties for a designer
to consider are the optical ones, including the transmission range, which describes
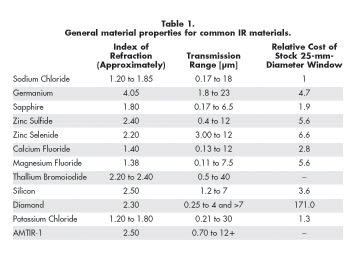
the wavelengths that the material can transmit. Transmission range information,
however – such as that included in Table 1 – considers only whether
some specific fraction of the incident energy is submitted, not whether the material
will completely transmit the incident energy. To better understand the material’s
ability to transmit it completely, look at a complete transmission curve, which
can be found in the manufacturer’s or supplier’s product information.
Another important quality is the refractive index (n), which dictates
a material’s ability to control and manipulate light. The ratio of the speed
of light in a vacuum to the speed of light in a different material, the refractive
index is a function of both the light’s wavelength and the temperature of
the material being considered. Typically, materials designed for use in the infrared
have higher n values, which allows for better correction of third- and higher-order
aberrations.1
A third and very important optical property is dispersion, the
derivative of the material’s refractive index with respect to wavelength,
or how the index of refraction changes as the wavelength changes. For transparent
materials, dispersion is typically a negative value. Dispersive properties of a
material can be a benefit or a drawback. The prism in a spectrometer, for instance,
needs dispersion to separate wavelengths. In lenses, however, having large amounts
of angular dispersion will cause chromatic aberrations and should be avoided. Thus,
dispersion must be considered based on the optic’s end use.

At top Edmund Optics’ germanium windows are available
uncoated or with two antireflection coating options: 3 to 12 μm for mid-IR
or broadband multispectral applications, or 8 to 12 μm for thermal imaging
applications. Below, the company’s mid-wave IR achromatic lenses are suitable
components for applications in Fourier transform IR spectroscopy and mid-wave IR
thermal imaging, and for use with tunable quantum cascade lasers.
As a result of the index of refraction being wavelength- and,
thus, frequency-dependent, the material’s molecular structure also can affect
optical performance. Consider the case where the material has a crystalline structure
that is anisotropic (directionally dependent). In this case, the material will cause
light to experience double refraction, a process in which incident light is split
into two refracted beams. One beam is known as the ordinary beam because it correctly
obeys the law of refraction; the other is referred to as the extraordinary ray because
it is refracted despite having a perpendicular incidence. A material that experiences
double refraction is considered to be birefringent. Birefringence is measured as
the difference in the index of refraction between the extraordinary rays and the
ordinary rays.2
Thermal properties
A common misconception for the IR region of the electromagnetic
spectrum is that these wavelengths transport heat; consequently, they are sometimes
referred to, incorrectly, as “heat waves.” In reality, the frequencies
of IR wavelengths either cause the vibrational modes of certain molecules to become
excited or the molecules themselves to rotate. Many materials that absorb the IR
also have rotational or vibrational resonances in these wavelengths. It is these
resonances that cause the materials to become hot.
Because most materials that work well optically in the IR do not
have 100 percent transmission and, consequently, absorb the IR, their vibrational
or rotational resonances can be excited, causing temperature changes within the
material. Thus, it is important to consider the thermal properties when choosing
an IR material.3 Table 2 shows the thermal properties of some common IR optical
materials.

The thermal conductivity (k) of a material describes how well
thermal energy is transmitted through it. Thermal conductivity is the measure of
heat that successfully passes through a unit thickness of material in a direction
normal to a surface of unit area resulting from a unit temperature gradient. The
thermal conductivity can be used to predict the amount of energy lost as light passes
through the system. As with refraction, thermal conductivity can be bidirectional,
as determined by the crystalline structure of the material.
If a material does not have unit thermal conductivity, some of
the energy is absorbed, and heat is created. As the heat is applied and absorbed
by the material, the internal temperature changes. The rate at which the temperature
changes depends on the material. The specific heat capacity (c) is defined as the
heat necessary to raise the temperature of a unit of mass of the substance by 1
°C. While the definition of specific heat capacity is uniform regardless of
the material being tested, when comparing materials, it is easier to look at specific
heat directly. The specific heat of a material is the ratio of the material’s
specific heat capacity to the specific heat capacity of water.
If the material undergoes changes in temperature, the material
properties can change significantly. This can cause significant alterations in the
optical properties. When a material is heated, through the absorption of vibrational
or rotational energy, the dimensions can change. The characterization of this dimensional
change is referred to as the material’s coefficient of thermal expansion.
The dimensional fluctuation of a material is one of the leading causes of focal
plane shifts for IR imaging systems; in most cases, this can be accounted for through
athermalization.1
Similar to the coefficient of thermal expansion, which simply
accounts for changes resulting from gradual temperature variations, it is important
to consider a material’s thermal stability, which is the measure of a material’s
ability to resist sharp or sudden changes in temperature without catastrophic material
failure. One last thermal property to consider when choosing an IR material is the
melting point, the temperature where a material changes physical states (for example,
from solid to liquid).
Mechanical properties
Because the environmental conditions of IR components are dependent
upon the end use, it is important to be cognizant of how the materials will behave
given some more rugged applications. In many cases, the mechanical properties can
serve as great decision points when the optical performances are similar. Table
3 summarizes key mechanical properties for common IR materials.
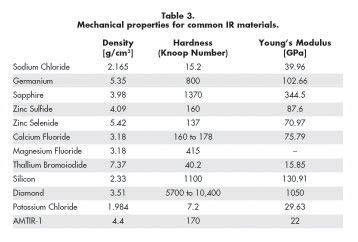
One important mechanical property is the specific gravity of the
material. Sometimes called relative density, it is the ratio of the material’s
density to the density of water. Although specific gravity is a unitless number,
numerically it is equivalent to the density when using metric units. As such, the
specific gravity is listed instead of the density in Table 3.
When making considerations around the ruggedness of a material,
look at the material’s hardness. There are several standard tests for hardness.
Here the Knoop hardness test is considered. The Knoop number is calculated by measuring
the indentation made on the surface of the test material after pressing a pyramidal
diamond point with a given load. The Knoop number for materials can vary from small
values for soft materials (4 kg/mm2 for potassium bromide) to high values for hard
materials (7000 kg/mm2 for diamond).
One final mechanical property to consider when choosing a material
for IR optics is the Young’s modulus, or the measure of the stiffness of the
material. Dealing specifically with elastic deformations, Young’s modulus
is the ratio of the stress (force per unit area perpendicular to the force) to the
strain, or the resulting fractional stretching (or compressing) of the material.
Material comparison
The wide variety of optical, thermal and mechanical properties
used to evaluate the fit for a material to be used in an optical design ultimately
means that the designer must perform a trade-off study. Unfortunately, the lack
of a single IR material reference guide can make this study difficult. Many designers
have found a temporary solution by keeping handbooks and reference guides on their
desks, with pages for their favorite IR materials bookmarked. Housing all this information
in one location will help designers focus on optical design rather than on researching
material properties.
Meet the author
David Henz is product line manager at Edmund Optics Inc. in Tucson,
Ariz.; e-mail: dhenz@edmundoptics.com.
References
1. A. Mann (2009). Lenses, infrared optics and zoom. Proc SPIE,
Vol. TT83, pp. 2-30.
2. P. Klocek et al (1991). Handbook of infrared optical materials.
Optical Engineering Series. Klocek, ed. Vol. 30. Marcel Dekker Inc., pp. 141-154
and 193-426.
3. E. Hecht (2002). Optics. 4th Ed. Addison Wesley, pp. 76-78.
/Buyers_Guide/Edmund_Optics/c4064